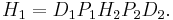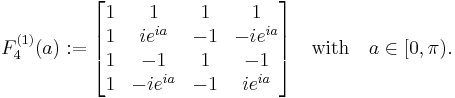Complex Hadamard matrix

A complex Hadamard matrix is any complex $N \times N$ matrix $H$ satisfying two conditions:

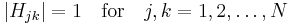
unimodularity (the modulus of each entry is unity): $|H_{jk}|=1 {\quad \rm for \quad} j,k=1,2,\dots,N$

orthogonality: $HH^{\dagger} = N \; {\mathbb I}$ ,

where 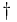${\dagger}$ denotes the Hermitian transpose of H and 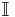${\mathbb I}$ is the identity matrix. The concept is a generalization of the Hadamard matrix.

Complex Hadamard matrices arise in the study of operator algebras and the theory of quantum computation. Real Hadamard matrices and Butson-type Hadamard matrices form particular cases of complex Hadamard matrices.

Complex Hadamard matrices exist for any natural N (compare the real case, in which existence is not known for every N). For instance the Fourier matrices

$[F_N]_{jk}:= \exp[(2\pi i(j - 1)(k - 1) / N] {\quad \rm for \quad} j,k=1,2,\dots,N$

belong to this class.

Equivalency

Two complex Hadamard matrices are called equivalent, written 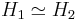$H_1 \simeq H_2$ , if there exist diagonal unitary matrices 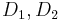$D_1, D_2$ and permutation matrices 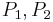$P_1, P_2$ such that

$H_1 = D_1 P_1 H_2 P_2 D_2.$

Any complex Hadamard matrix is equivalent to a dephased Hadamard matrix, in which all elements in the first row and first column are equal to unity.

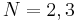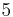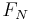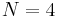For $N=2,3$ and $5$ all complex Hadamard matrices are equivalent to the Fourier matrix $F_{N}$ . For $N=4$ there exists a continuous, one-parameter family of inequivalent complex Hadamard matrices,

$F_{4}^{(1)}(a):= \begin{bmatrix} 1 & 1 & 1 & 1 \\ 1 & ie^{ia} & -1 & -ie^{ia} \\ 1 & -1 & 1 &-1 \\ 1 & -ie^{ia}& -1 & i e^{ia} \end{bmatrix} {\quad \rm with \quad } a\in [0,\pi) .$

For $N=6$ the following families of complex Hadamard matrices are known:

a single two-parameter family which includes $F_6$ ,
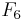a single one-parameter family $D_6(t)$ ,
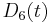a one-parameter orbit $B_6(\theta)$ , including the circulant Hadamard matrix $C_6$ ,
a two-parameter orbit including the previous two examples $X_6(\alpha)$ ,
a one-parameter orbit $M_6(x)$ of symmetric matrices,
a two-parameter orbit including the previous example $K_6(x,y)$ ,
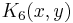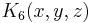a three-parameter orbit including all the previous examples $K_6(x,y,z)$ ,
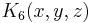a further construction with four degrees of freedom, $G_6$ , yielding other examples than $K_6(x,y,z)$ ,
a single point - one of the Butson-type Hadamard matrices, $S_6 \in H(3,6)$ .

It is not known, however, if this list is complete, but it is conjectured that $K_6(x,y,z),G_6,S_6$ is an exhaustive (but not necessarily irredundant) list of all complex Hadamard matrices of order 6.

References

U. Haagerup, Orthogonal maximal abelian *-subalgebras of the n×n matrices and cyclic n-roots, Operator Algebras and Quantum Field Theory (Rome), 1996 (Cambridge, MA: International Press) pp 296-322.
P. Dita, Some results on the parametrization of complex Hadamard matrices, J. Phys. A: Math. Gen. 37, 5355-5374 (2004).
F. Szollosi, A two-parametric family of complex Hadamard matrices of order 6 induced by hypocycloids, preprint, arXiv:0811.3930v2 [math.OA]
W. Tadej and K. Zyczkowski, A concise guide to complex Hadamard matrices Open Systems & Infor. Dyn. 13 133-177 (2006)

External links

For an explicit list of known $N=6$ complex Hadamard matrices and several examples of Hadamard matrices of size 7-16 see Catalogue of Complex Hadamard Matrices